Teaching with Materials

Counting Board

The Counting Board allows children to fit colored blocks into grooves, so children become familiar with the structure of numbers even before they are introduced to the number names or symbols. All pieces on the Counting Board are removable, allowing the teacher to provide the appropriate level of scaffolding for students while focusing on each concept within ten.

The Counting Board is used to teach:

• Understanding quantity
• Sequencing
• Counting
• Relationships between numbers (more and less, before and after, between)
• Number names
• Numerals (number symbols)

Discovering Size Relationships

Children discover size relationships and the structure of numbers within ten while completing the “puzzle” of the Counting Board. Even before learning the number names, students learn to match the size of the block to its groove. They discover that each block has its place in the sequence, and they learn the meaning of bigger, biggest, smaller, smallest. An important part of this stage is helping children begin to reason about numbers, construct arguments and critique those of others with the support of concrete manipulatives. These are important early concepts that must be mastered in order to reason meaningfully about numbers.

Learning to Count - Identifying Blocks by Their Number Names

When being introduced to the Counting Board children progress through the same sequence as they do in learning to talk. First, meaning is developed through actions and the perception of visual relationships; then in later experiments, the number names are introduced and used to identify the blocks. The children rapidly learn the number names and associate them with quantities represented by the blocks.

Introducing Number Symbols 1 to 10

The number symbols from 1 to 10 are introduced by attaching the number guide to the counting board and asking the children to match the symbols on the markers with the identical symbol on the number guide.

Learning to Associate the Number Block with the Number Symbol

Children indicate their recognition by drawing a line from the block to its symbol.
(A page from Structural Arithmetic Workbook I.)

Number Relationships

Temple Ary, math specialist and teacher trainer, working with first grade children at Harlem Success Academy in New York City. Temple is using the Counting Board to teach the numbers 1 to 10 and to show what numbers mean. The children can see the pattern of how the numbers increase by a single unit, the difference in length and height, the order of the numbers, and their relationship to one. She has not yet introduced the written symbols for the numbers because she wants the children to get these number sense concepts first.

Pattern Boards

By presenting a discrete number of 1-blocks carefully arranged in an organized structure, the Pattern Boards invite children to discern the relationship between numbers. For example, the difference between odd and even is readily apparent. This difference is not evident to the child when he or she is presented with a random array of cubes or any other set of objects. The Pattern Boards are a concrete representation of what is commonly referred to as “the ten frame.”

The Pattern Boards are used to teach:

• Counting
• Quantity
• Subitizing
• Number names
• Odd and even patterns
• One and two more/less
• Doubles and neighbors within 10
• Addition and subtraction within 10
• Problem solving within 10

The following sketches are from Experimenting with Numbers.

Naming the Pattern Boards and Putting Them in Sequence

Children are asked to recognize whether they have the Pattern Board that will hold the block pattern displayed by the teacher. Through this and other activities, students expand and generalize their understanding of quantity and sequence, learning the names of the different Pattern Boards in the same way they learned the block names.

Learning about Even Number Patterns

The concept of even numbers is demonstrated by placing the pattern boards in sequence from 1 to 10. The children then observe that the even patterns are built from pairs of 1-blocks which are partners.

Learning to Write the Symbol 8

In this workbook page (fromStructural Arithmetic Book I) children learn to write the symbol 8 and to study the quantity for which it stands and the place it comes in the sequence of even numbers.

Even Numbers

Temple Ary with second graders at Harlem Success Academy in New York City, using the Pattern Boards to teach odd and even. When the units are displayed on the 10 grid, it is much easier to see the pattern of the single units in the odd and even numbers. The Structural Arithmetic program introduces numbers and their patterns in different ways so that children get a thorough understanding. Here the numbers are broken up into single units. In the counting board they are represented in a length model.

10-Box

The 10-Box can be used to teach and reinforce the sequence of numbers by building a staircase from 1 to 10. This tool can also be used to teach addition, subtraction and problem solving within 10. Additionally, students use the 10-box to practice their combinations of 10 through activities and games.

Learning the Combinations that Make 10- Finding Missing Addends

Students learn to find the missing addend in combinations of 10. The teacher places a block in the 10-box and asks the child to find the block that goes with it to be as big as 10.

From Experimenting with Numbers.

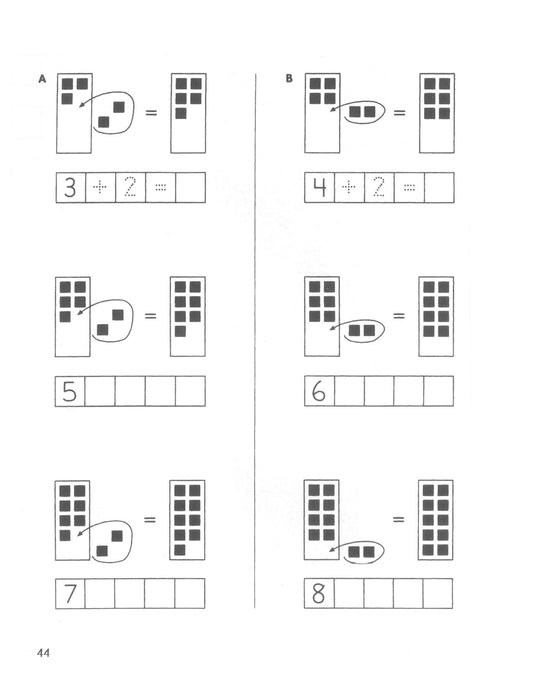
Recording Addition Facts

In this workbook page, children are asked to write the missing addend in the blanks. They draw on their knowledge of the blocks which went together in the 10-box.

From Structural Arithmetic Book I.



Number Box 1 to 10

These Number Boxes for numbers 1-10 model the concept of hierarchical inclusion as well as the fact-families for each number up to 10. Early on, students can be challenged to put the blocks in order, using size relationships to create the “staircase” for each number without the scaffolding provided by the Counting Board. Children are encouraged to verbalize their thinking. They realize that the number 5 is made up of smaller numbers 1-4 (hierarchical inclusion) and that there are many ways to make each number. Children discover that only even numbers can be represented by two of the same size block (doubles facts).

The Number Boxes are used to teach:

• Sequencing
• Hierarchical inclusion
• Fact families for the numbers up to 10
• The relationship between addition and subtraction
• Fact fluency within 10
• Problem solving with addition and subtraction within 10

Discovering all the combinations to make a number

Children discover that there are many ways to make a number and all the patterns in those combinations: each block appears twice, providing a visual presentation of the associative principle (“turn-around” facts), and the inverse relationship between the two addends (one staircase goes up while the other side goes down).

From Experimenting with Numbers.

Equation Template

The Equation Template allows young children to translate into symbolic form what they are discovering about the combinations of numbers. They become acquainted with the meaning of the symbols and the structure of an equation. Equivalence is simultaneously represented in its concrete and abstract forms.